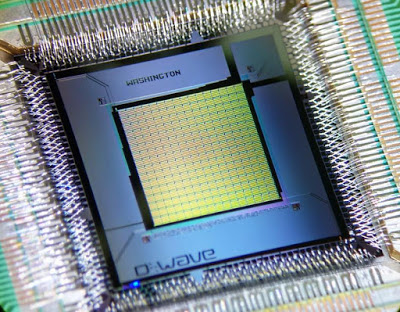
A critical question for the field of quantum computing in the near future is whether quantum devices without error correction can perform a well-defined computational task beyond the capabilities of state-of-the-art classical computers, achieving so-called quantum supremacy. We study the task of sampling from the output distributions of (pseudo-)random quantum circuits, a natural task for benchmarking quantum computers.

Crucially, sampling this distribution classically requires a direct numerical simulation of the circuit, with computational cost exponential in the number of qubits. This requirement is typical of chaotic systems. We extend previous results in computational complexity to argue more formally that this sampling task must take exponential time in a classical computer.

We study the convergence to the chaotic regime using extensive supercomputer simulations, modeling circuits with up to 42 qubits - the largest quantum circuits simulated to date for a computational task that approaches quantum supremacy. We argue that while chaotic states are extremely sensitive to errors, quantum supremacy can be achieved in the near-term with approximately fifty superconducting qubits.